Johannes Kepler's Polyhedra

    Johannes Kepler (1571-1630), best known for his three laws of planetary motion, was one of the most outstanding mathematicians of his day.  In addition to his astronomical accomplishments, he systematized and extended all that was known about polyhedra in his time.  While previous artist/geometers discovered particular polyhedra, he took a more mathematical approach: he defined classes of polyhedra, discovered the members of the class, and proved that his set was complete. For example, Kepler discovered the infinite class of antiprisms.

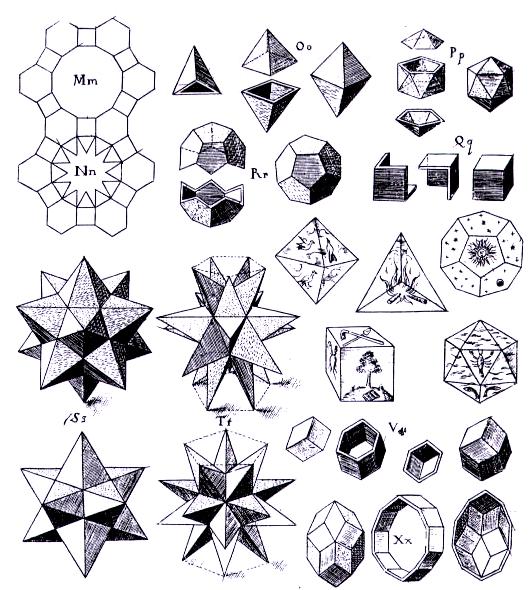
    Kepler's logical approach to polyhedra does not mean that he was free of the mysticism of the day.  The following illustration, from his 1619 book, Harmonice Mundi, graphically shows the Platonic associations of the regular solids with the classical elements: The tetrahedron corresponds to fire, the octahedron to air, the cube to earth, the icosahedron to water, and the dodecahedron to the cosmos or ether:

    The lower-left portion of the above figure illustrates Kepler's two star polyhedra, the small stellated dodecahedron and the great stellated dodecahedron.  Although earlier illustrations of these solids exist, Kepler was the first to recognize them as meeting the definition of regular polyhedra, but with nonconvex pentagram faces. (An earlier mosaic of the small stellated dodecahedron is attributed to Uccello and an earlier drawing of the great stellated dodecahedron appears in the work of Jamnitzer.) Kepler saw them from a deeper perspective, and in recognition, we now refer to these as Kepler solids.

    The lower-right portion of the above figure illustrates the disassembly of two rhombic solids which Kepler discovered: the rhombic dodecahedron and the rhombic triacontahedron.  (An  illustration of an approximation to the triacontahedron is clear in one of Jamnitzer's monuments drawn fifty years earlier, but Kepler certainly had a much deeper understanding of the triacontahedron's structure and its relationships to other polyhedra.)  Below, left, Kepler shows how a dodecahedron can be constructed by adding "roofs" to the six sides of a cube (the construction which Euclid uses).  Below, right, is how Kepler constructs a rhombic dodecahedron analogously.  These figures are from his book Epitome of Copernican Astronomy.

     

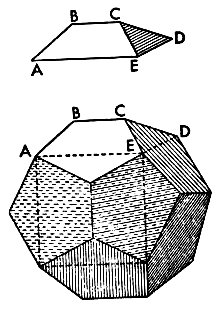
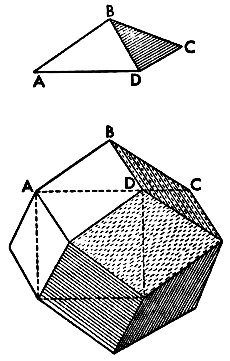
    In, Harmonice Mundi, Kepler also defined the class of convex uniform polyhedra which we call the Archimedean solids, unfamiliar with the fact that Archimedes had defined the class already.  All of these polyhedra except one (the snub dodecahedron, number 13 at the bottom right below) had already appeared in the work of various Renaissance artists.  But those artists were haphazard, and wrote as if there could be an infinite number of related polyhedra which they were selecting from. Kepler's important contribution was to define this class of polyhedra and systematically explore it, to find all its members and prove his set was complete. In doing so, he realized that the prisms and antiprisms belong to the same class, since at each vertex the same combination of regular polygons meet.

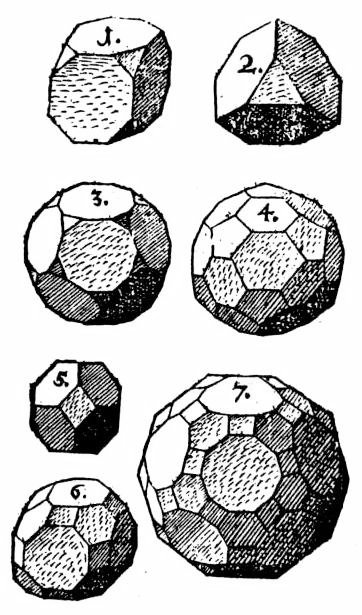
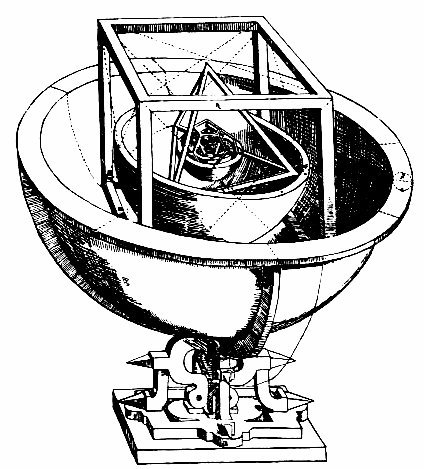
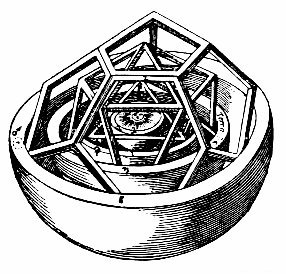
    In my view, a most artistic contribution of Kepler is to be found in his model of the solar system.  Kepler proposed that the distance relationships between the six planets known at that time could be understood in terms of the five Platonic solids.  His 1596 book, Mysterium Cosmographicum, proposed the model illustrated below, in which one Platonic solid fits between each pair of planetary spheres.  (Note the use of Leonardo's style of open faces.)
    The outer sphere is that of Saturn; inside it is the sphere of Jupiter.  Kepler made two prototypes in colored paper hoping to have it fabricated in silver. His original plan was that it would also function as a punchbowl dispensing assorted beverages.

    The image at right is a closeup of the spheres of inner planets, Mercury, Venus, Earth, and Mars.  This is a beautiful astronomical model.   For example, it explains why there are only six planets:  How could there be a seventh planet, when Euclid proved that there are only five Platonic solids!  Of course, the model is completely false, the interplanetary distances it predicts are not sufficiently accurate, and Kepler was scientist enough to accept this eventually. But it an excellent example of how truth and beauty are not always equivalent.

    Virtual Polyhedra, (c) 1998, George W. Hart