Polyhedra with Intersecting Faces

    If you have never before looked at polyhedra in which the faces intersect, this may give you a kind of AHA! experience. The initial reaction of many people who view these objects is to focus on locally connected surfaces. But, to understand these polyhedra, you must see larger connections which may take a minute before they jump out at you. The color scheme used here is important.

    Here are three examples to illustrate:

    1) The faces here pass through each other, but the colors indicate how the visible parts connect, even when parts of a face are hidden behind other faces. For example, this rhombidodecadodecahedron contains blue squares and green pentagons partially hidden behind each other and blue-green pentagrams, but no triangles (which would have been red). Study it until you see it as made only of large squares, pentagons, and pentagrams. Looking at it while it is slowly rotating brings this out most clearly. Each of the "real" vertices is the meeting of a pentagram, a square, a pentagon, and another square. The other apparent corners are not real vertices, just places where edges happen to cross each other.



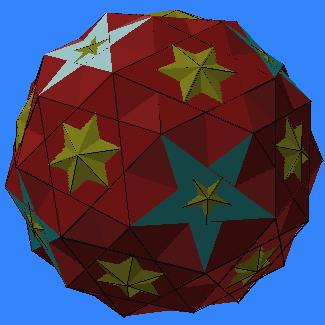    2) As another example, there are triangles in this compound of the snub disicosidodecahedron and its dual, but they are not the little red triangles as you might at first think; those are just parts of larger triangles. All the "real" triangles are quite large, having an edge long enough to go all the way across a pentagram. This polyhedron also shows that two polygons may happen to lie in the same plane. What looks like a red six-pointed star is really two co-planar triangles.



    3) The yellow in the above figure is bits of the faces from the dual polyhedron, the hexagonal hexecontahedron, peeking out. This is what it looks like alone; its faces are all identical irregular hexagons. Color won't help here; you just have to see the planes. The middle of each face is hidden behind a pyramid formed by three other faces. I have included black lines where two faces of the same color meet, to help distinguish the borders of the polygons from lines where the planes happen to cross.



    By the way, these are all good examples to fly inside of. Try to imagine the shape of their interiors before going in to look. (1) and (2) have quite interesting innards. Also, find where the yellow pyramids are tucked in behind the red triangles in (2). Can you find a polyhedron in this collection whose outside is the same as (3)'s inside ?

    Exercise: Count how many triangles there are in this small triambic icosidodecahedron.

    Answer: Twenty, not sixty.

    Exercise: Keeping in mind how large the triangles really are, imagine what you can see from the center of this polyhedron.

    Answer: Go inside and see if you were right.


    Virtual Polyhedra, (c) 1996,George W. Hart