Holyhedra !
John
Conway has proposed the problem of finding "holyhedra," meaning every face
contains a hole. Here are some constructions of mine.
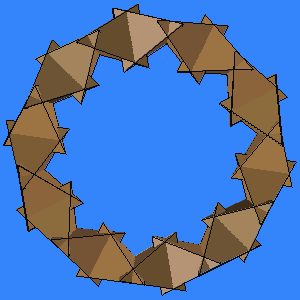
The thing at right is constructed using twelve tetrahedra. Each face
contains one hole, strictly interior to it. However, each face is disconnected,
as a tip in each face plane is separated from the rest. Since a disconnected
face is not usually considered a face, this is more of an "unholyhedron."
This mostly serves as an example to make one be precise about the the definitions.
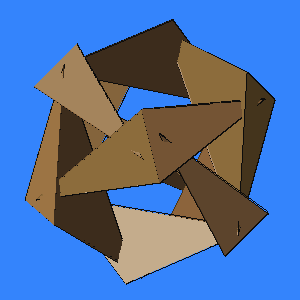
Another
unholyhedron, at left, made from twenty tetrahedra, has no disconnected
faces, but the holes touch the edges of the face at two points.
As of this writing, no one has yet found a holyhedron in which each
face is connected and has a strictly interior hole (i.e., the hole doesn't
touch the outer edge of the face.)